Self-inductance and LR circuit

1. Self-induced emf's

Consider a loop or coil carrying a current i. Then it exists, in the vicinity of the loop, an induced magnetic field B given by the Biot-Savart law. The induced field is proportional to the current i. The changing magnetic flux Φ linking the loop produces the emf given by Faraday's law ℰ = - dΦ_B/dt. We obtain a varying flux by moving a magnet. But the varying magnetic flux can be also obtained by varying B then by varying the current i. This process of changing the current in a circuit (loop's or coil's own current) produces a self-induced emf.

If the magnetic field B induced is proportional to i, then does the magnetic flux. We write Φ = L i

Faraday's law becomes

ℰ_L = - dΦ_B/dt = - L di/dt

The constant of proportionality L is defined as the self-inductance (or inductance) for the loop.

The SI unit of inductance in the Henry (H). It is a large unit of inductance. the values of self-inductance of coils in electronic circuits are typically in the 1µH to a mH range.

2. Example of a solenoid

Solenoid of length l, and cross-sectional area S with n turns per unit length. The magnitude of the field is B = μ_o n i. The flux linking one loop is Φ_B = μ_o n i S. The flux linking a solenoid is NΦ_B , where N = nl.
The emf induced in the N-turn coil solenoid is ℰ_L = - N dΦ_B/dt = - nl μ_o n S di/dt

Therefore

L = μ_on²Sl

L = μ_on²Sl

That is the self-inductance of an ideal (infinitely long) solenoid, where we neglect the end effects.

The inductance can be measured in a circuit that contain the related element as a coil.

3. L R circuit

3.1. Switch at the position 1

At the position 1, the battery is connected; the current increases during a certain time until it stabilizes and becomes equal to the steady value I = ℰ_o/R.



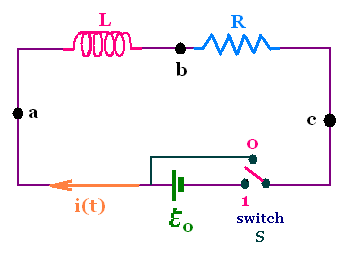
Consider a circuit with a coil or solenoid providing a self-inductance connected to a battery through a switch. When the switch is closed, the battery causes charge to move in the circuit. The solenoid has a self-inductance L and a resistance R.

As the current i(t) in the circuit changes through the switch, there is a self-induced emf ℰ_L = - L di(t)/dt in the inductance that opposes a change in the current, preventing it to vary abruptly. The instantaneous value of the current depends on the value of L, R and the emf of the battery ℰ_o.

We apply the loop rule: Starting at the point a, proceeding clockwise, and equating the sum of the voltage change to zero gives:

(V_a - V_c) + (V_b - V_a) + (V_c - V_b) = 0

We have:

V_a - V_c = ℰ_o
V_b - V_a = - L di/dt
V_c - V_b = - R i

Therefore:

ℰ_o - L di/dt - Ri = 0
- L di/dt = Ri - ℰ_o = R(i - ℰ_o/R)
We obtain the following differential equation:
di/(i - ℰ_o/R) = - (R/L )dt
Integrating, we obtain:
i(t) = Const. exp { - (R/L)t} + ℰ_o/R
Where Const is a constant integration.

We find this constant Const by applying the initial condition i(0) = 0:
At t = 0, i(0) = 0 = Const + ℰ_o/R

Thus

Const = - ℰ_o/R

We define a characteristic time parameter: τ_L = L/R called the inductive time constant.
i(t) = - (ℰ_o/R) exp {- t/τ_L} + ℰ_o/R

i(t) = (ℰ_o/R) (1 - exp {- t/τ_L})

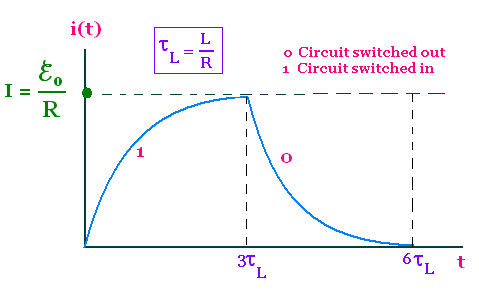
At a long time (t → ∞) i(t) becomes equal to the steady value I = ℰ_o/R, that is the value of the current i without the inductance. The inductive time constant τ_L = L/R sets the time scale for the LR circuit. At t = 5τ_L, the value of the current i(t) is already 0.99 ℰ_o/R.

3.1. Switch at the position 0

At the position 0, the battery is disconnected; the current decreases during a certain time until it vanishes, and becomes equal to the steady value I = 0.

At the time t = 0, while the current has the value i_o, we disconnect the battery. Thus the loop rule, with ℰ_o is :

0 - L di/dt - Ri = 0.

Solving this differential equation, using the initial condition i(0) = i_o, gives:

i(t) = i_oexp {- t/τ_L}

At the time t = τ_L, the current has dropped to i_oexp {- 1} = 0.37i_o. At t = 3τ_L, the current becomes practically zero.

4. Energy transfers in LR circuits

4.1. Energy stored in an inductor

The gravitational potential energy of a mass changes as the mass moves through a gravitational field.

The electric potential energy of charge carriers changes as carriers move through a potential difference in a circuit element.

Consider an inductor, of ohmic resistance r and inductance L. In addition to the Joule heating in the inductor at the rate P_r = ri², there is also an electric potential energy U_L due the self-induced emf ℰ.

If the current is increasing in the inductor, the self-induced emf opposing the current is negative, hence the carriers lose potential energy.

If the current is decreasing in the inductor, the self-induced emf opposing the current is positive, hence the carriers gain potential energy.

The potential energy lost by the charge carriers when the current is increasing is maintained within or stored in the inductor due to the change in the potential difference between the conductor.

The potential energy gained by the charge carriers when the current is decreasing is returned or restored or recovered by the inductor due to the change in the potential difference between the conductor.

Each time the potential energy if transformed to and from a stored energy in the inductor, called the magnetic energy of the inductor.

The power P, or rate of energy used or transformed in the inductor is P = dU_L/dt, where dU_L is the infinitesimal electric potential energy change stored in the inductor. This change dU corresponds to the change di in current.

We have P = dU_L/dt = ℰi = i L (di/dt)

P = Li(di/dt)

Since dU_L/dt = i L (di/dt), or

dU_L = L i di, we integrate to obtain

U_L(t) = (1/2) L i²(t) + const.

At t = 0, U_L(t) = 0. Hence const = 0.

Therefore the energy stored in the inductor is:

U_L = (1/2) L i²

4.1. Energy stored in an inductor

Consider a solenoid of length l, and cross-sectional area S with n turns per unit length. Its inductance is: L = μ_on²Sl. When crossed by a current I, the produced magnetic field is B = μ_onI.

Therefore

U_L = (1/2) L i² = (1/2) μ_on²Sl i² =
(1/2) μ_on²Sl (B/μ_on)² =
(1/2) Sl B² (1/μ_o) = Sl B²/2μ_o

The product Sl represents the volume of the space inside the solenoid. Hence the energy density stored in the solenoid is u_B = U_L/V = B²/2μ_o