Quantum Mechanics
Schrodinger equation
Quantum Mechanics
Propagators : Pg
Quantum Simple Harmonic
Oscillator QSHO
Quantum Mechanics
Simulation With GNU Octave
© The scientific sentence. 2010
|
| Dirac equation: free particle
1. Dirac's equation
The main idea is to change the D'Alembertian operator in Klein_Gordon
equation into a Lorentz invariant.
Here is Klein-Gordon equation is:
(1/c2)∂2/∂t2ψ - ∇2ψ + (mo2c2/ħ2)ψ = 0
or
☐ψ + (moc/ħ)2ψ = 0
where
☐ = (1/c2)∂2/∂t2 - ∇2
Like a 4-Vector, the wave function ψ(r,t) for which will be applied
the square root of the D'Alembertian must have 4 components. Therefore,
this square root of the D'Alembertian will be transformed by matrices.
Dirac factorised the d'Alembertian as follows:
∇2 = - (1/c2)∂2/∂t2
= (A∂/∂x + B∂/∂y + C∂/∂z + (i/c)D∂/∂t)
(A∂/∂x + B∂/∂y + C∂/∂z + (i/c)D∂/∂t)
The cross-terms of the operator A, B, C, and D will vanish, and their
square must be equal to the operator indentity I. That is:
Their anticommutators {X,Y} = 0 and X2 = Y2 = I.
(X and Y are any operator A, B, C, or D).
Hence, the Klein_gordon equation:
☐ψ + (moc/ħ)2ψ = 0
becomes:
[∇2 - (1/c2)∂2/∂t2]ψ = (moc/ħ)2ψ
Or
(A∂/∂x + B∂/∂y + C∂/∂z + (i/c)D∂/∂t) ψ = (moc/ħ) ψ
Let's set:
A = iβα1, B = iβα2 , C = iβα3, and D = β
The above equation becomes:
iβ [(α1∂/∂x + α2∂/∂y + α3∂/∂z + (1/c)∂/∂t)] ψ = (moc/ħ) ψ
Using the symbol ∇ of the gradient operator with
the vector matrix α = (α1, α2, α3), we get:
iβ [α.∇ + (1/c)∂/∂t)] ψ = (moc/ħ) ψ
Multiplying the both sides by ħc, we get:
[iβħc α.∇ + iħ β ∂/∂t)] ψ = (moc2) ψ
Since β2 = 1, we have:
[iħc α.∇ + iħ∂/∂t)] ψ = (βmoc2) ψ
Using the quantum expression of momentum operator P = -iħ∇, we get:
[- c α.P + iħ∂/∂t)] ψ = (βmoc2) ψ
We find finally the equation:
(iħ∂/∂t) ψ = (cα.P + βmoc2) ψ
That is the Dirac equation.
Dirac equation:
(iħ∂/∂t) ψ = (c α.P + βmoc2) ψ
α1, α2, α3, and β are 4x4 matrices.
2. Dirac's matrices
Dirac defined the matrices α1, α2,
α3, and β as the following:
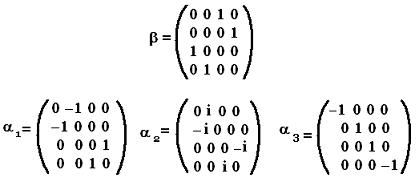
2. γ-Dirac's matrices
More better, new matrices, known as the gamma matrices are defined:
γ0 = β
γi = β αi,
i = 1, 2, 3.

Dirac Equation becomes;
(iħγ0∂/∂t) ψ = (c γ.P + moc2) ψ
(iħγ0∂/∂t) ψ = (c γ.P + moc2) ψ
Using the Pauli's matrices:
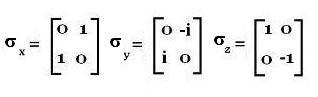
we can write:

|
|