Calculus I
Limits
Derivative
Exercices
Applications
Marginal analysis
© The scientific sentence. 2010
|
Calculus I: Derivative applications
Ohmic circuit
Ohmic circuit
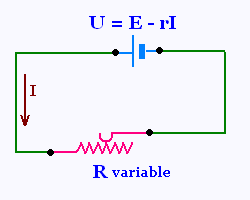
Let's consider an electric circuit including a battery
and an exterior variable resistance (resistor) R.
The battery has a constant emf = E, and an interior constant
resistance r.
a) What is the value of the resistor R to have a max
power in the circuit?
U increases at the rate of 0.5 volt/sec, while I
decreases 0.1 amps/sec. t is the time in seconds,
b) find an equation relating dR/dt dV/dt and dI/dt
c) find dR/dt when V = 9 volts and I = 3 A.
We known that U = E - rI and U = IR. Where I is the intensity of
the electric current in the circuit, and U is the voltage.
The know also that P = U I = RI2. Where P is the electric power
of the circuit. So
U = RI = E - rI. Then
E = (r + R)I or I = E/(r + R)
P = U I = RI2 = R (E/(r + R))2 =
E2 x R/(r + R)2 =
P = E2 R/(r + R)2
The maximum of P is obtained by taking its
derivative equal to zero. That is : dP/dR = 0.
dP/dR = E2 ((r + R)2 - 2R(r + R))/(r + R)4 =
E2 (r2 + 2rR + R2 - 2Rr - 2R2)/(r + R)4 =
E2 (r2 - R2)/(r + R)4 =
E2 (r + R)(r - R)/(r + R)4 =
E2(r - R)/(r + R)3
dP/dR = E2(r - R)/(r + R)3
dP/dR = 0 yields r = R. Hence
The power is maximum in the R-circuit when the interior
resistance is equal to the exerior resistance.
b) U = RI. Taking the differential yields:
dU/dt = R dI/dt + I dR/dt. Since U = RI, one has
dU/dt = (U/I) dI/dt + I dR/dt
c) dR/dt = (1/I) (dU/dt - (U/I) dI/dt) =
(1/3) (0.5 - (9/3) x 0.1) = (0.5 - 0.3)/3 = 0.067.
dR/dt = 0.067 Ω/sec.
dR/dt = 0.067 Ω/sec.
|
|

