Calculus III
Contents
3 Dimensional space
Partial derivatives
Multiple integrals
Vector Functions
Line integrals
Surface integrals
Vector operators
Applications
© The scientific sentence. 2010
|
|
Calculus III:
Triple integrals in caresian coordinates
The formula for the general triple integrals is:
∫∫∫E f(x, y, z) dV
where
dV = dx dy dz
1. Triple integrals in caresian coordinates
over a box : rectangular regions
Consider the box E = B = [a,b] x [c,d] x [r,s], where are listed
the x�s first, the y�s second and the z�s third.
The triple integral in this case is:
∫∫∫B f(x, y, z) dV =
∫rs
∫cd
∫ab
f(x, y, z) dx dy dz
Note that we integrated with respect to x first, we iterate
then for y, and finally for z.
Notice that this order is not important. There are 6 different possible
orders to do the integral and that give the same result regardless of the order.
It is a matter of convenience and doing the calculus in the easiest manner.
2. Examples
Example 1
Conmpute
∫∫∫B 24 x y z dV
over the box B = [1,2] x [0,1] x [2,3].
The triple integral is:
∫∫∫B f(x, y, z) dV =
∫23
∫01
∫12
24 x y z dx dy dz =
24 ∫23
∫01
∫12
x dx y z dy dz =
24 ∫23
∫01
(x2/2)|12 y z dy dz =
12 ∫23
∫01
(4 - 1) y z dy dz =
36 ∫23
∫01
y dy z dz =
18 ∫23
y2|01 z dz =
18 ∫23
1 z dz =
18 ∫23
z dz =
9 z2|23 =
9 (9 - 4) = 45 . Therefore
∫∫∫B 12 x y z dV = 45
Example 2
Conmpute
∫∫∫E dV
over the box B = [a,b] x [c,d] x [r,s].
The triple integral is:
∫∫∫EdV =
∫rs
∫cd
∫ab
dx dy dz =
(b - a)(d - c)(s - r). That is
the volume of the box of sides (b - a), (d - c), and (s - r).
In the general case, the volume of the three-dimetional region E is given by:
V = ∫∫∫E dV
3. Triple integrals in general three-dimensional regions
1. Along the z-axis
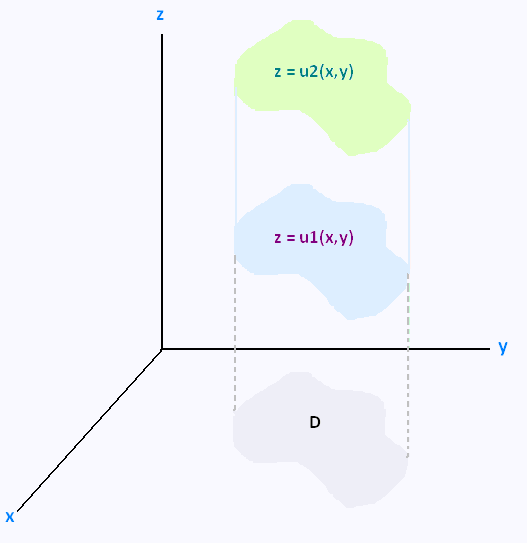
The region E is defined as :
E = {(x,y,z)|(x,y) ∈ D, u1(x,y) ≤ z ≤ u2(x,y)}
The triple integral is:
∫∫∫E f(x,y,z) dV =
∫∫D
∫u1(x,y)u2(x,y) f(x,y,z) dz dA
R(x,y) = ∫u1(x,y)u2(x,y) f(x,y,z) dz
The double integral ∫∫D R(x,y) dA can be evaluated in any of
the methods that we have seen.
Example 1
Compute
∫∫∫E f(x, y, z) dV .
Where E is the region under the plane 3x + 5y + z = 15 ,
that lies in the first octant.
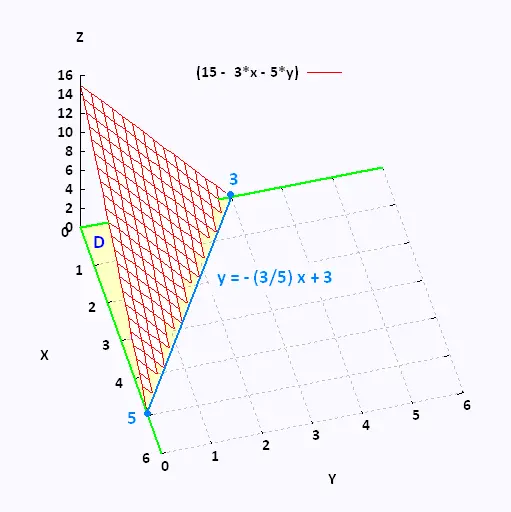
The region D lies in the xy-plane. It is the triangle
with vertices at (0,0), (5,0), and (0,3).
Here are the limits of integration:
The region E is located in the first octant. So it is
above the plane z = 0.
0 ≤ z ≤ 15 - 3x - 5y
0 ≤ x ≤ 5
0 ≤ y ≤ - (3/5) x + 3
Or :
0 ≤ x ≤ - (5/3) y + 5
0 ≤ y ≤ 3
Therefore, the integral is:
∫∫∫E f(x,y,z) dV =
∫∫D
∫0 15 - 3x - 5y f(x,y,z) dz dA
=
∫∫D
R(x,y) dA
=
∫05
∫0- (3/5) x + 3
R(x,y) dy dx
=
∫05
Q(x) dx
= Real value.
2. Along the x-axis
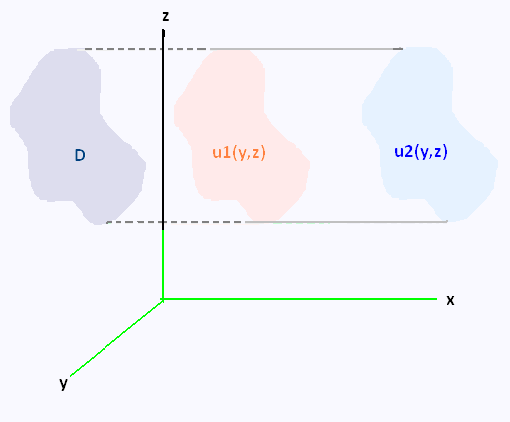
The region E is defined as :
E = {(x,y,z)|(y,z) ∈ D, u1(y,z) ≤ z ≤ u2(y,z)}
The triple integral is:
∫∫∫E f(x,y,z) dV =
∫∫D
∫u1(y,z)u2(y,z)) f(x,y,z) dz dA
R(y,z) = ∫u1(y,z)u2(y,z) f(x,y,z) dz
The double integral ∫∫D R(y,z) dA can be evaluated in any of
the methods that we have seen.
Example 2
Compute
∫∫∫E dV .
Where E is the region under the plane 2x + y + z = 8 ,
delimited by z = 3√y and z = 3y/2.
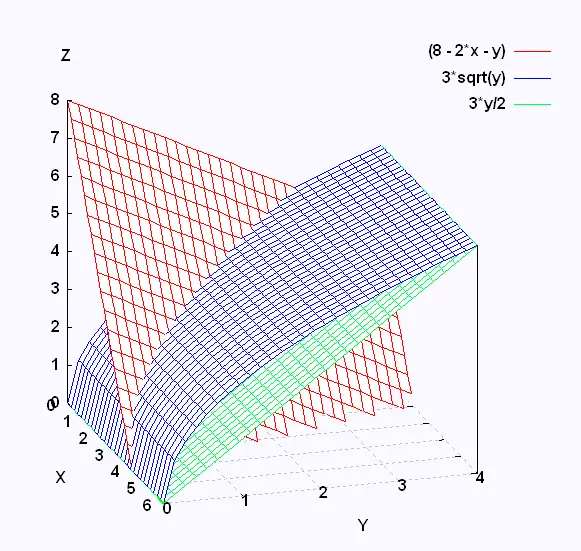
Here are the limits of integration:
3y/2 ≤ z ≤ 3√y
0 ≤ x ≤ (8 - y - z)/2
0 ≤ y ≤ 4
Therefore, the integral is:
∫∫∫E dV =
∫∫D
∫0(8 - y - z)/2 dx dA
∫04
∫3y/23√y
(8 - y - z)/2 dz dy
=
∫04
R(y) dy
= Real value.
3. Along the y-axis
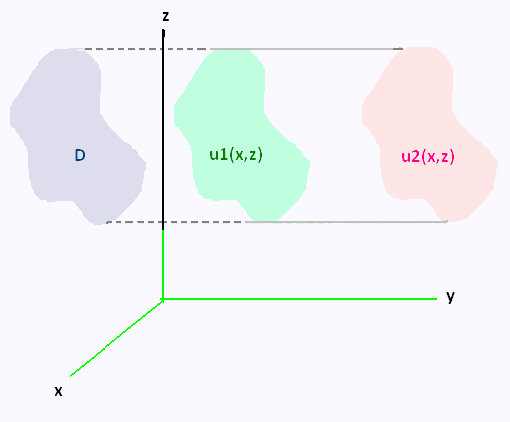
The region E is defined as :
E = {(x,y,z)|(x,z) ∈ D, u1(x,z) ≤ z ≤ u2(x,z)}
The triple integral is:
∫∫∫E f(x,y,z) dV =
∫∫D
∫u1(x,z)u2(x,z)) f(x,y,z) dy dA
=
∫∫D
R(x,z) dA
The double integral ∫∫D R(x,z) dA can be evaluated in any of
the methods that we have seen.
Example 3
Compute
∫∫∫E f(x,y,z) dV .
Where E is the solid bounded by y = 4x2 + 4z2,
and the plane y = 8.
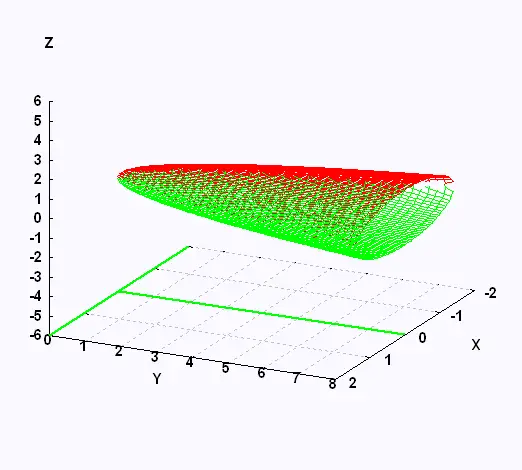
The disk at y = 8 has the equation:
4x2 + 4y2 = 8 → x2 + z2 = 2, then
the radius of √2.
In polar coordiantes, we have:
x = r cos θ z = r sin θ
Here are the limits of integration:
4x2 + 4y2 ≤ y ≤ 8
0 ≤ r ≤ √2
0 ≤ θ ≤ 2π
Therefore, the integral is:
∫∫∫E dV =
∫∫D
∫4x2 + 4y2 8 f(x,y,z) dy dA
∫0 2π
∫0 2√2
R(x,z) r dr dθ
= Real value.
Gnuplot:
Example 1:
----------
reset
set ticslevel 0
set grid
set xtics 1
set ytics 1
set ztics 2
set hidden3d
set isosamples 30,30
set xzeroaxis lt 2 lw 2
set yzeroaxis lt 2 lw 2
set style line 3 lw 3
set xrange [0:6]
set yrange [0:6]
set zrange [0:16]
set ylabel "Y"
set xlabel "X"
set zlabel "Z"
splot (15 - 3*x - 5*y) lw 1
Example 2:
----------
reset
set ticslevel 0
set grid
set xtics 1
set ytics 1
set ztics 1
set hidden3d
set isosamples 30,30
set xzeroaxis lt 2 lw 2
set yzeroaxis lt 2 lw 2
set style line 3 lw 3
set xrange [0:6]
set yrange [0:4]
set zrange [0:8]
set ylabel "Y"
set xlabel "X"
set zlabel "Z"
splot (8 - 2*x - y) , 3*sqrt(y), 3*y/2
Example 3:
----------
reset
set grid
set xtics 1
set ytics 1
set ztics 1
set ticslevel 0
set isosamples 80,40
set xzeroaxis lt 2 lw 2
set yzeroaxis lt 2 lw 2
set style line 3 lw 3
set xrange [-2:2]
set yrange [0:8]
set zrange [-6:6]
set ylabel "Y"
set xlabel "X"
set zlabel "Z"
splot sqrt(y - 4*x**2)/2, - sqrt(y - 4*x**2)/2
|
|