Calculus III
Contents
3 Dimensional space
Partial derivatives
Multiple integrals
Vector Functions
Line integrals
Surface integrals
Vector operators
Applications
© The scientific sentence. 2010
|
|
Calculus III:
Vector functions
Vector fields
Gradient vector field
Conservative vector fields
1. Definition
A vector field is a function vector  that assigns to each point
M in the space a vector (M).
In two-dimension space,
M(x,y): (M) = (x,y).
In three-dimension space,
if M(x,y,z):(M) = (x,y,z).
The standard notation of a vector field (M)
is built as :
• In two-dimention space:
(M) = P(x,y)  + Q(x,y,z) 
• In three-dimention space:
(M) = P(x,y,z) + Q(x,y,z) + R(x,y,z)
2. Example
Let's sketch vector field (M) =
- (x/2) + (y/2)
To graph the vector field, let's do evaluations.
• (0, 0) =
• (1, - 1) = - (1/2) - (1/2)
At the point (1, - 1), we plot the vector
- (1/2) - (1/2).
• (- 4, - 2) = 2 - 1
At the point (- 4, - 2), we plot the vector
2 - .
(1, 2) =
- (1/2) + 1.
At the point (1, 2), we plot the vector
- (1/2) + .
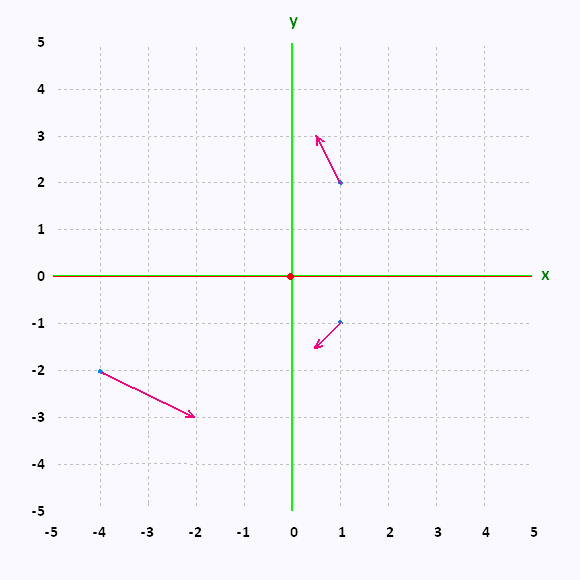
We can continue in this fashion plotting vectors for several points and we’ll get an almost complete sketch of the vector field.
3. The special case: The gradient vector
The special case of vector field function is the the gradient vector.
Given a function f(x, y, z), the gradient vector is written
∇f , and defined by:
∇f = fx +
fy +
fz
Where
fx = ∂f/∂x,
fy = ∂f/∂y, and
fz = ∂f/∂z,
Notice that f(x, y, z) is called scalar function .
For a two-variable function f(x,y), we just need to drop off the third component of the vector.
4. Conservative vector fields
A vector field is called a conservative vector field if there exists a function ƒ such that = ∇ƒ.
If is a conservative vector field then the function, Æ’ is called a potential function for
.
That is, a vector field is conservative if it is a gradient vector field for some function.
Exemple
The vector field = y + x is a conservative vector field with a potential function of Æ’(x, y) = xy.
Indeed ∇ƒ = 〈 y, x 〉.
|
  |