Calculus III:

Vector functions (t)
Definitions and graphs

1. Defnitions

A vector function is a function that takes one or more variables and returns a vector.

We’ll consider vector functions of a single variable and vector functions of two variables.

A vector function of a single variable in and have the following respective forms:

(t) = (f(t), g(t)) and

(t) = (f(t), g(t), h(t))

(f(t), g(t), h(t)) are the component functions.

t is a real parameter.

The first component function gives the x coordinate, the second component function gives the y coordinate, and the third component function gives the z coordinates of the point that we graph.

2. Examples

(t) = (2sin(t), √(x - 5), √(3 - x)) is a vector function. What is its domain?

The domain of a vector function is the set of all t’s for which all the component functions are defined.

The first component 2sin(t) is defined over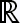, in the second component √(x - 3): x ≥ 3, and in the third √(5 - x): x ≤ 5. Hence the domain is the interval [3, 5].

3. Graphing a vector function

Example 1

Graphing a vector function consists of drawing the position vectors for each point on the graph. The a position vector , say(a, b, c) , is a vector that starts at the origin and ends at the point (a, b, c).

The method to graph a vector function is to choose values of the parameter t to get the components of the corresponding vector position we get out of the vector function.

Sketch the graph of the vector function (t) = (t, 2)

Some values of t give us some position vectors:

(-4) = (- 4, 2), (-1) = (-1, 2), (0) = (0, 2), and (3) = (3, 2).

Then the points (- 4, 2), (-1, 2), (0, 2), and (3, 2) are all on the graph of this vector function.

Here is a sketch of this vector function:


The graph of the vector function(t) = (t, 2) is a line .

Example 2

Sketch the graph of the vector function (t) = (2t, t² - 3)

Some values of t give us some position vectors:

(-3) = (- 6, 6), (-1) = (-2, -2), (0) = (0, -3), and (2) = (4, 1).

Then the points (- 6, 6), (-2, -2), (0, -3), and (4, 1) are all on the graph of this vector function.

Here is a sketch of this vector function:

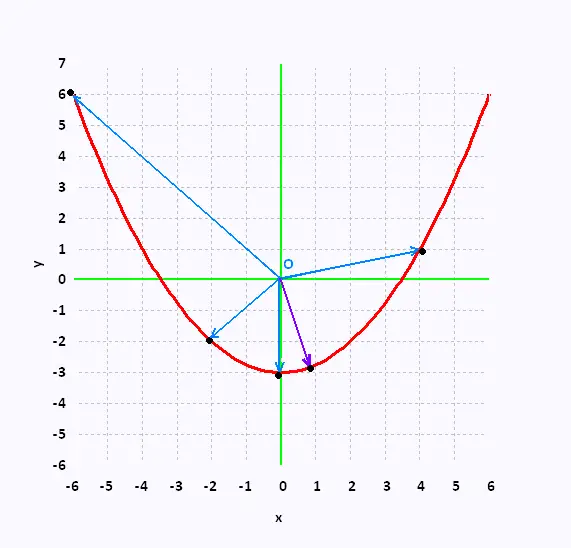
The graph of the vector function (t) = (2t, t² - 3) follows the graph of the second component t² - 3, that is an hyperbola.

Example 3

Sketch the graph of the vector function (t) = (2*cos(t), sin(t))

Some values of t give us some position vectors:

(0) = (2, 6), (π/2) = (0, π/2), (π) = (- 2, 0), and (3π/4) = (0, -1).

Then the points (2, 6), (0, π/2), (- 2, 0), and (0, -1) are all on the graph of this vector function.

Here is a sketch of this vector function:


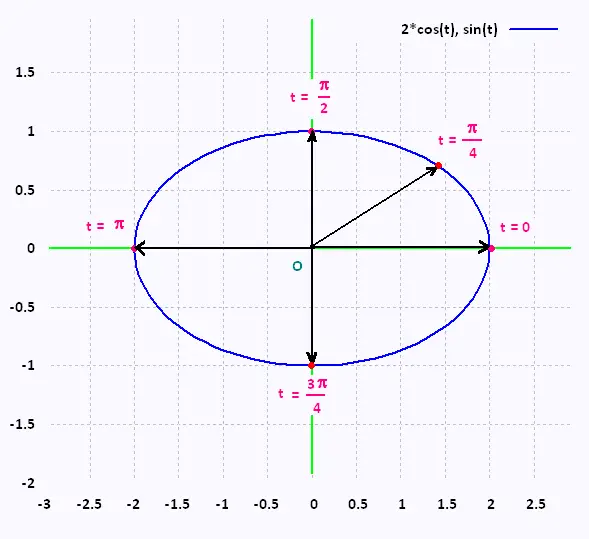
The graph of the vector function(t) = (2*cos(t), sin(t)) is an ellipse.

4. vector functions in R³

The three dimensional vector function(t) = (f(t), g(t), h(t)) can be broken down into the parametric equations = x = f(t), y = g(t), and z = h(t).

Examples

1) The graph of the vector function(t) = (1 + 3t, - 2 + t, - 5 - 2t) is a line that passes by the point (1, -2, -5), and having = (3, 1, - 2) as the parallel vector.

2) The graph of the vector function(t) = (3sin(t)), 3cos(t), 4) gives a circle, of radius 3, centred at the origin, parallel to the xy-plane and located at z = 3.

3) Likewise, the graph of the vector function(t) = (3sin(t)), 4, 3cos(t)) gives a circle, of radius 3, centred at the origin, parallel to the xz-plane and located at y = 4.

4) The graph of the vector function(t) = (3sin(t)), 3cos(t), t) gives a helix (or spiral), of radius 3, centred at the origin, parallel to the xz-plane along the z-axis.

5) The graph of the vector function(t) = (3sin(t)), t, 3cos(t)) is a helix that rotates around the y-axis, and the graph of the vector function(t) = (t, 3sin(t)), 3cos(t)) is a helix that rotates around the x-axis.