|
Electrostatics
Electromagnetics
Electricity &
Magnetism
© The scientific sentence. 2010
|
Electrostatics:
Effect of the field on a charged particle.
1. Free charged particle in a electric field
We are considering the simplest situation where the
electric field is uniform .
In an electric field E, if the only significant
force on a charged particle q is the electric force
F, then the Coulomb's law and Newton second law together
give the expression of the net force:
F = q E = m a or a = q E/m
a is the acceleration of the charged particle of
mass m.
1. A charged particle released in a uniform electric
field E will be accelerated a long the electric field.
At the origin, we place the release point and orient
the field parallel to the x-axis.
The kinematics of the particle released from rest
(vo = 0), like a free-fall particle, is:
ax = q E/m
x = (1/2)axt2 = (1/2)(qE/m)t2
vx = axt = (qE/m)t
ax = q(E/m)
x = (1/2)axt2 = (1/2)(qE/m)t2
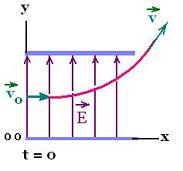
2. Projectile charged particle in a electric field
Now if the positive charged particle enters the field E oriented
along the y-axis, perpendicularly
with an initial velocity vo at t = 0, like
a projectile, its kinematics equations are:
ax = 0
ay = qE/m
x = vot
vx = vo
vy = ayt = (qE/m)t
x = vot
y = (1/2) ayt2 = (1/2) (qE/m)t2 =
(1/2) (qE/m)(x/vo)2 =
(1/2) (qE/m)(1/vo)2. x2
That is the particle follows the parabolic path.
vx = vo
vy = ayt = (qE/m)t
x = vot
y = (1/2) ayt2 = (1/2) (qE/m)t2 =
(1/2) (qE/m)(x/vo)2 = (1/2) (qE/m)(1/vo)2. x2
|
|