Relativity: The 4-Vectors

1. Lorentz Transformations

From the reference frame at rest (RF):
The velocity of the reference frame (RF) is 0
The velocity of the moving reference frame (MF) is v
The velocity of the moving object is u.

From the moving frame (MF):
The velocity of the moving reference frame (MF) is 0
The velocity of the reference frame (RF) is - v
The velocity of the moving object is u'


In the (MF):
Galilean transformations give:

t' = t
x' = x - vt
y' = y
z' = z


and
Lorentz Transformations give:

t' = γ(t - vx/c²)
x' = γ(x - vt)
y' = y
z' = z


where
γ = 1/[1 - β²]^1/2, and β = v/c

We have also the reverse transformation:

t = γ(t' + vx'/c²)
x = γ(x' + vt')
y = y'
z = z'

2. Proper time

If an object is at rest in the mooving reference frame u' = 0. So its velocity in the (RF) is v, because the velocity of the (MF) is v in the (RF).

In the (RF):
If the object starts to move at t = 0 along the x-axis. At a time later t, the object is located at:
x = vt, y = 0, z = 0, t = t

In the (MF):
The object is located at:
x' = γ(vt - vt) = 0
y' = y
z' = z
t' = γ(t - v vt /c²) =
γ t (1 - (v /c)²) = γ t (1/γ²) = t/γ

In the (RF): time = t, and
In the (MF): time = t' = t/γ < t
In the (RF) t = γ t' : The time is dilated.

t' is the proper time, which measures time in the moving reference frame.

3. 4-Vectors notations

For an event E = (t, x, y, z), Lorentz transformation is then an operator that transforms this 4-Vector on another 4-Vector event E' = (t', x', y', z') = (γ(t - vx/c²), γ(x - vt), y, z) within a certain vector space called the Minkowski space, which is a 4-Dimension Cartesian space with a specific property of the inner product.

But, in order to have the same dimension, that is a length, for all of the 4 components, we just make following change in Lorentz transformations, and get:

ct' = γ(ct - vxc/c²) x' = γ(x - vtc/c)
y' = y
z' = z


That is:
ct' = γ(ct - βx) x' = γ(x - β ct)
y' = y
z' = z


And have instead:
E = (ct, x, y, z), transformed in:
E' = (ct', x', y', z') = (γ(ct - βx), γ(x - β ct), y, z)

4. The invariant (Δs)² = - c²t² + r²

In the (MF):


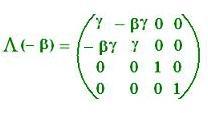
The event E = (ct, x, y, z), transformed into the event:
E' = (ct', x', y', z') = (γ(ct - βx), γ(x - β ct), y, z),

ct' = γ(ct - βx) x' = γ(x - β ct)
y' = y
z' = z

Therefore
E' = Λ(- β) E

In the (RF):


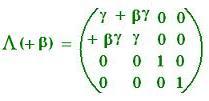
The event E' = (ct', x', y', z'), is transformed in the event:
E = (ct, x, y, z) = (γ(ct' + βx'), γ(x' + βct'), y', z')

ct = γ(ct' + βx') x = γ(x' + β ct')
y = y'
z = z'

Therefore

E = Λ(+β) E'

That we can write:
c(-t') = γ(c(-t') - βx')
x = γ(x' - β c (-t'))
y = y'
z = z'


So, in the (MF):
The event E' = (-ct', x', y', z'), is transformed in the event:
E = (-ct, x, y, z) = (γ(-ct' - βx'), γ(x' - βct'), y', z')

Therefore

E = Λ(-β) E'

That is:

If E is tranformed with Λ(-β) in E', E' is tranforme with Λ(+β) in E. Thus, to transform E' in E with Λ(-β), we have to change the time t' into -t'. That is we replace (ct, x, y, z) = Λ(+β) (ct', x', y', z') by (-ct, x, y, z) = Λ(- β) (-ct', x', y', z')

Hence:
(ct', x', y', z') = Λ(-β) (ct, x, y, z)
(-ct, x, y, z) = Λ(- β) (-ct', x', y', z')

or

(ct, x, y, z) = Λ^{- 1}(ct', x', y', z')
(-ct, x, y, z) = Λ(- β) (-ct', x', y', z')


The scalar product gives:
(ct, x, y, z) . (-ct, x, y, z) = Λ^{- 1}(ct', x', y', z') . Λ(- β) (-ct', x', y', z') = (ct', x', y', z') (-ct', x', y', z')

or
(ct, x, y, z) . (-ct, x, y, z) = (ct', x', y', z') (-ct', x', y', z')

That is
- c²t² + x² + y² + z² = - c²t'² + x'² + y'² + z'²

Therefore, if the vector space r has the components x, y , and z:

Under Lorentz transormations, the squared norm of an event is constant: The squared distance: - c²t² + x² + y² + z² = - c²t² + r² is an invariant .

(Δs)² = - c²t² + r² is invariant .

5. Covariance and contravariance Notations

We will use the following notation:

For the kind of 4-Vector: (ct, x, y, z):
(ct, x, y, z) = (x⁰, x¹, x², x³) or x^μ for short, μ = 0, 1, 2, 3.

x^μ is called contravariant 4-vector.

For the kind of 4-Vector: (- ct, x, y, z):
(- ct, x, y, z) = (x₀, x₁, x₂, x₃) or x_μ for short, μ = 0, 1, 2, 3.

x_μ is called covariant 4-vector.

So

(Δs)² = x_μ . x^μ = Σ x_μ x^μ
μ = 0, 1, 2, 3.
= x₀x⁰ + x₁x¹ + x₂x² + x₃x³

The fact that to use x_μ for short, leads to following notation called Einstein notation:
Σ x_μ x^μ = x_μ x^μ
μ = 0, 1, 2, 3.

Let's find again that the defined scalar product is invariant:
(a⁰, a) = a^μ un event, Lorentz-transformed into (a'⁰, a') = a'^μ, and
(b₀, b) = b_μ un event, Lorentz-transformed into (b'₀, b') = b'_μ

Remark that
b_μ = (b₀, b) = (- b⁰, b)

a'^μb'_μ = (a'⁰, a')(b'₀, b') = - γ(a⁰ - βa¹)γ(b⁰ - βb¹) + γ(a¹ - β a⁰)γ(b¹ - β b⁰) + a²b² + a³b³ =

γ²[ - a⁰b⁰ + βa¹b⁰ βa⁰b¹ + βb¹a⁰ - βb¹βa¹ + a¹b¹ - β a⁰b¹ - β b⁰a¹ + β b⁰β a⁰] + a²b² + a³b³

= γ²[ (a⁰b⁰ - a¹b¹) (β² - 1)] a²b² + a³b³ =

- a⁰b⁰ + a¹b¹ a²b² + a³b³

= a^μb_μ

Therefore

a'^μb'_μ = a^μb_μ = invariant

Remark:
a^μb_μ = (a⁰, a)(b₀, b) =
(a⁰, a)(- b⁰, b) = (- a⁰, a)(b⁰, b) =
= ( a₀, a)(b⁰, b) = a_μb^μ

Therefore

a^μb_μ = a_μb^μ