Rotational dynamics: Atwood's machine

1.Atwood's machine 3

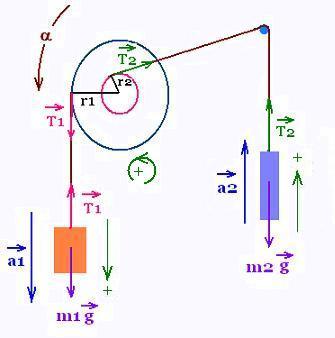
A pulley of moment of inertia I is made of two disks of radii r1 and r2, welded together. The masses m1 and m2 are attached to two strings, and each string is wound around one of the disks as shown in the figure.

2. Second Newton's law for rotation

The second Newton's law for rotation for the pulley is:
τ_net = Στ = I α    (1)
That is :

T1 r1 - T2 r2 = I α    (1')

α is the angular speed of the pulley. This angular speed α is the same for the two disks because they are attached to each other.

At the static equilibrium (for rotation and translation), the angular speed is constant or zero (system at rest). Then: α = dω/dt = 0. Therefore, the formula (1') becomes:
T1 r1 - T2 r2 = I 0 = 0, then T1 r1 = T2 r2

The two torques τ1 = T1 r1 and τ2 = T2 r2 are equal when the system is at rest or at constant speed.

The tangential acceleration "a1" for the disk 1 is
a1 = α r1    (2)
And
The tangential acceleration "a2" for the disk 2 is
a2 = α r2    (3)

3. Second Newton's law for translation

The second Newton's law for translation of the two masses is:
For m1:
m1 g - T1 = m1 a1    (4)

For m2:
- m2 g + T2 = m2 a2    (5)

From (4):
T1 = m1 g - m1 a1 = m1 (g - a1)    (4')

From (5):
T2 = m2 g + m2 a2 = m2(g + a2) (    (5')

4. Solving for the tensions and accelerations

Multiplying (4') by r1 and (5') by r2, and using the formula (1') yields:
T1 r1 - T2 r2 = r1 m1 (g - a1) - r2 m2(g + a2) = I α

Using the relationships (2) and (3), we get:

T1 r1 - T2 r2 = r1 m1 (g - α r1) - r2 m2(g + α r2) = I α

Developing:

T1 r1 - T2 r2 = r1 m1 g - α m1r1² - r2 m2 g - α m2r2²

Rearranging to solve for α leads to:

r1 m1 g - r2 m2 g - α (m1r1² + m2r2²) = I α
r1 m1 g - r2 m2 g = α (m1r1² + m2r2² + I)

Therefore:

α = (r1 m1 g - r2 m2 g)/(m1r1² + m2r2² + I)

We have then:

α = g(r₁ m₁ - r₂ m₂)/(m₁r₁² + m₂r₂² + I)
a1 = α r1
a2 = α r2
T1 = m₁(g - a₁)
T2 = m₂(g + a₂)

5. Applications

m1 = 3 kg, m2 = 1 kg
r1 = 10 cm , r2 = 5 cm
I = 0.20 kg.m²
g = 9.8 m/s²

α = 9.8(0.10 3 - 0.05 1)/(3 (0.10)² + 1 (0.05)² + 0.20) =
10.54 rad/s²

a1 = α r1 = 10.54 0.10 = 1.05 m/s²

a2 = α r2 = 10.54 0.05 = 0.53 m/s²

T1 = m₁(g - a₁) = 3(9.8 - 1.05) = 26.25 N

T2 = m₂(g + a₂) = 1 (9.8 + 0.53) = 10.33 N