Dynamics
Contents
Applications
Exercises
© The scientific sentence. 2010
| Dynamics: Frictions within two blocks
on inclined plane
Newton's law applications
1. Frictions: Two blocks on inclined plane
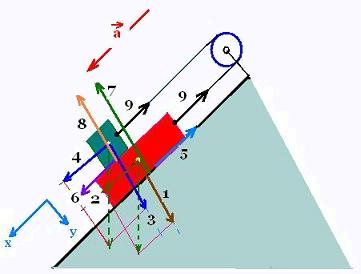
1: is the projection of the weight M2g over the axis y = M2g cosθ
2: is the projection of the weight M2g over the axis x = M2gsinθ
3: is the projection of the weight M1g over the axis y = M1g cosθ
4: is the projection of the weight M1g over the axis x = M1gsinθ
5. f1 :is the friction force of the block (M1 + M2) under the support
6. f2: is the friction force of M1 under M2 and vise-versa
7: is the normal force of the mass M2
8. is the normal force of the mass M1
9. is the tension over the string on the pulley
a: is the acceleration of the system ( block = M1 + M2),
μk: is the kinetic Friction coefficient.
g: is the acceleration of gravity.
We suppose that
the two parts of the system are made up from the same substance,
2. The acceleration of the system
f1 = (M1 + M2)cosθ gμk (1)
f2 = M2cosθ gμk (2)
M1 g sin θ - T - f1 - f2 = M1 a (3)
M2 g sin θ - T + f2 = - M2 a (4)
(3) - (4) gives:
[M1 - M2]g sin θ - f1 - 2f2 = [M1 +M2] a
a = [(M1 - M2)g sin θ - (f1 + 2f2)] / [M1 + M2]
f1 + 2f2 = (M1 + 3 M2)cosθ gμk
a = [(M1 - M2)g sin θ - (M1 + 3 M2)cosθ gμk] / [M1 + M2]
a = [(M1-M2)gsin θ - gμkcosθ(M1+3M2)]/(M1+M2)
|
|