1. Definitions

The original statement of the Newton's third law is:

Law III. To every action there is always opposed an equal reaction.

That is: whenever two bodies interact, the two forces that they exert on each other are always equal in magnitude and opposite in direction.

Newton's third law applies to an interaction between two objects, whereas the first and second Newton's laws apply to a single object.

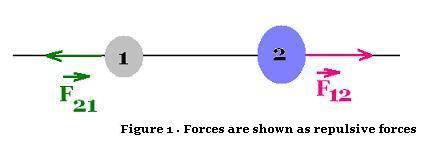
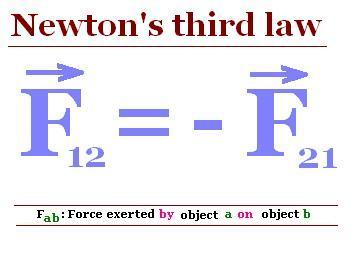
If object 1 exerts a force F12 on object 2, then , necessarily, object 2 exerts a force F21 on object 1. Newton's third law states that these two forces are equal and opposite, or

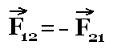
These two forces are equal in magnitude and opposite in direction. One is called action force, the other is called reaction force. The two forces F_ab and F_ba form an action-reaction pair.

2. Force symbol F_ab

Note that forces occur in pairs. A single force cannot exist.

Two subscripts a and b are required on the force symbol F_ab. The first subscript a denotes the object that exerts the force, and the second subscript b denotes the object on which the force acts.

The action-reaction pair is indicated by the reversal of the subscripts on the forces symbols.

3. Examples

3.1. Normal force

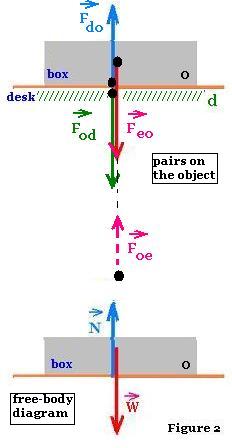
The force N is called the normal force and the force W is called the weight of the object o.

Is the couple (N,W) an action-reaction pair?

N is the force exerted by the desk d on the object o: F_do, wheras W is the force exerted by the earth on the object o: F_eo.

We dont have a reversal of subscripts, therefore the two forces N and W do not constitute an action-reaction pair.

These two forces are equal and opposite, without forming an action-reaction pair.



The two forces in the Newton's third law never occur in the same free-body diagram. This is because a free-body diagram shows forces acting on a single object.

3.2. Solved example: Many objects

Suppose that two objects are placed on a floor, with the object 2 on top of the object 1 (figure 3). We want to determine the reaction of the floor on the object 1.

3.2.1. Forces acting on the two objects

All the five forces are represented for the two objects.
Three for the object 1:

1. F_e1: The weight of the object 1, that is the force exerted by the earth on the object1.
2. F_f1: The force exerted by the floor on the object1.
3. F₂₁: The force exerted by the object 2 on the object 1, because there is an interaction between them,

and two forces for the object 2:

1. F_e2: the weight of the object 2, that is the force exerted by the earth on the object2.
2. F₁₂: The force exerted by the object 1 on the object 2, that is the reaction for the action F₂₁ (or the action of the reaction F₂₁).

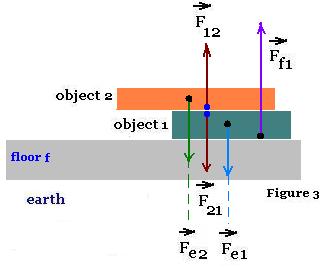
All the forces acting on the objects 1 and 2 are drawn. Among these forces, we have one action-reaction pair: (F₂₁, F₂₁).
Note that Newton's third law applies to an interaction between two objects.

3.2.2.Related free-body diagrams

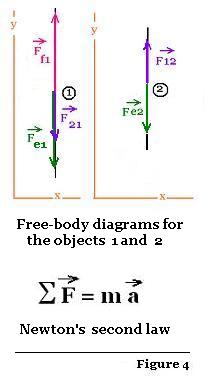
Now, to apply Newton's second law, we need to separate the objects, and draw a free-body diagram for each object alone . A free-body diagram shows forces acting on a single object.

object1:

The vector sum of the three forces is:
F_e1 + F_f1 + F₂₁ = m₁ a₁
Where m₁ and a₁ are the mass and the acceleration of the object 1 respectively.

Since the object 1 is at rest, we have a₁ = o; therefore
F_e1 + F_f1 + F₂₁ = 0. Thus:

F_f1 = - F_e1 - F₂₁

object2:

The vector sum of the two forces is:
F_e2 + F₁₂ = m₂ a₂ = 0, because a₂ = 0. Thus:

F₁₂ = - F_e2

If the object1 weighs 8.00 Newtons and the object 2 weighs 10.0 Newtons, what is the magnitude of the reaction of the floor on the object 1?

F_f1 = 18.00 Newtons.

4. Exercices

4.1. Newton's third law and friction

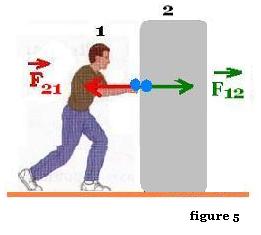
Explain why the person is able to push the box despite Newton's third law F₁₂ + F₂₁ = 0.

Hint:
What will happen when there is no friction on the floor?

4.2. Free fall motion and Newton's third law

Explain why the earth does not move toward an abject undergoing a free fall motion, according to the Newton's third law. The interaction between the two objects is shown in the figure 6.

Hint:
Use Newton's second law. The mass of the earth is M_e = 5.97 x 10²⁴ Kg, and the acceleration of gravity has the value g = 9.8 m/s².