Atom in magnetic field

A plane circular current loop of radius r, then of area πr; carrying an electric current I, has a magnetic dipole of magnetic moment vector μ = I A; where A is the vector area normal to the area A.

Once this magnetic dipole is placed in a magnetic field B, this field exerts a torque τ = μ x B on the dipole doing a work on it corresponding to a potential energy U = - μ . B, in order to align its field to the magnetic moment.

We want to apply this results to electron in Hydrogen atom, using the results of the Bohr atom; and therefore show that the orbital angular momentum L alone of the lectron is not sufficient to explain anmalous Zeeman effect.

1. Electron orbital angular momentum

1.1. The Bohr magneton

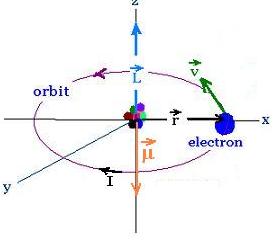
We consider the electron in the Bohr atom rotating about an axial (z-axis) passing through the nucleus (proton) as a circular loop carrying one electron per revolution.

The elecron is of magnitude charge e, mass m, tangential speed v, orbital angular speed ω angular momentum L, and orbits with a period T on a circle of radius r

We have:
ω = v/r = 2π/T
I = e/T
A = πr²

Therefore:
μ = I A = (e/T) πr² = (e v/2πr) πr² = e v r/2

Using the definition of the angular momentum L = mvr yields:
μ = e v r/2 = eL/2m

Because the electron charge is negative, the orbital angular momentum and magnetic moment vectors are opposite

The ratio of the magnitude of μ and L is constant and equal to μ/L = e/2m. It is called gyromagnetic ratio. Using the quantization of the angular momentum : L = mvr = n ħ, yields:
μ = eL/2m = e n ħ/2m

For n = 1, (ground state), the electron orbits on the first layer, hence the related magnetic moment is called Bohr magneton . Its magnitude is: μ_B = e ħ/2m; and its value is 9.274 x 10^{- 24} J/T (or A.m²).

1.2. The correct formula of the magneton

Bohr model gives an expression of the gyromagnetic ratio μ/ħ = e/2m for the ground state n = 1.



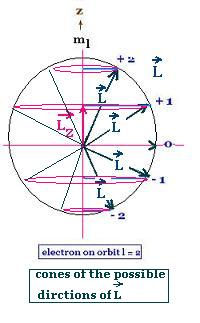
But the Schrodinger results for a hydrogen atom show that the quantization of the angular momentum must be written:

L = [l(l + 1)]^1/2ħ, and
L_z = m_lħ; the projection (component) of L on the axial z-axis.

where:
l is the orbital quantum number with 0 <= l <= n - 1, and
n is the principal quantum number with n >= 1
m_l is the magnetic quantum number with |m_l| <= l

The angular momentum L and the magnetic moment μ used by Bohr must be replaced by their components projection on the axial z-axis. Also, the quantum number n used by Bohr must be replaced by m_l, so:

L_z = m_lħ
μ_z = m_l eħ/2m

Replacing the magnitude charge by its actual charge, we have: μ_z = m_l eħ/2m

The Schrodinger formulation predicts for the ground state with m_l = 0 a zero magnetic moment, a value excluded by the Bohr's model.

Now, if the atom is present in a magnetic field B directed along the z-axis, the interaction of this field with the magnetic moment μ gives rise to the torque *tau; that corresponds to a potential energy for the field U = μ · B = - μ_z B = m_l eħB/2m . That is the orbital magnetic interaction energy:

2. The effect of the magnetic field on the orbital state of the electrom

The electron rotating on an orbit about z-axis crossing the nucleus has a (quantum) mechanic angular momentum L and, because it is charged, a corresponding orbital magnetic moment μ. L and μ are always antiparallel. The quantization of L leads to set that its z-component L_z = m_lħ. For a principal quantum number n, we have (n - 1)n/2 values of l; and for each l, we have (2l + 1) values of m_l. The energy of the electron depends on its principal quantum number only, that is E_n = - 13.6 eV/n². Therefore, without a magnetic field, all the (n-1)n/2 x (2l+1) states would have the same energy E_n, that is all these states would be degenerate.

For an orbital state l, we have (2l + 1) values of m_l, then (2l + 1) values of μ_z, and (2l + 1) values of L_z. All these (2l + 1) states are shown and split from each other by a magnetic field. The effect of the magnetic field is to remove this degeneracy, that is to split the energy of each orbital state by an amount of U =m_l μ_BB.

In the presence of a magnetic field, the energy of an electron in the state l, will be split into (2l + 1) distinct energy levels. Two adjacent levels are separated by the energy μ_B B.

Example:

For a hydrogen atom at the state n = 2, the electron is either in the orbital state "s" (l=0) or the orbital state "p" (l=1).
we have l = 0 and l = 1.

No magnetif field:
E₂ = -13.6/4 = - 3.4 eV

With magnetic field:
If the electron orbits in the state "s", then: l = 0 : m_l = 0
E = - 3.4 eV + 0

If the electron orbits in the state "p", then: l = 1 : m_l = -1, 0, +1
E = - 3.4 eV - μ_B B
E = - 3.4 eV + 0
E = - 3.4 eV + μ_B B

3. Larmor precession

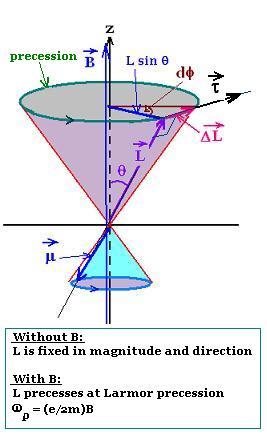
The atom is placed in an external magnetic field B directed along the axial z-axis. The magnetic moment μ (proportional to the orbital angular momentum L: μ_z = - (e/2m)L) undergoes a torque parallel to ΔL = μ x B. This torque, perpendicular to the orbital angular mmentum L, tends to line up the magnetic moment with the magnetic field B along the z-axis. Therefore, the torque exerted produces a change in orbital angular momentum dL which is perpendicular to that angular momentum L, according to the law: τ = dL/dt. This change in orbital angular momentum causes the magnetic moment to precess around the direction of the magnetic field. This is called Larmor precession.

If θ is the angle where L is fixed, and φ the precession angle, we have:
τ = ΔL/Δt = dL/dt = (L sinθ) dφ/dt
Since τ μ x B = μ B sin θ, and μ = (e/2m) L
Therefore:
μ B sin θ = (L sinθ) dφ/dt, then:
μ B = L dφ/dt, or dφ/dt = (B/L)μ = (e/2m) B

Setting ω_p = dφ/dt, the expression of the Larmor precession is: