Statics

1. Massless string

A horizontal force F acts on a block A and moves it to the left. The block B is connected to the block A by a massless string. The whole system: "block A + string + block B" moves to the left at an acceleration a.

The free body diagram for the string gives according to Newton's second law:
T_AonS - T_BonS m_s a
where m_s is the mass of the string.

If the string is massless, then: m_s = 0,
Therefore:
T_AonS = T_BonS    (1)

According to the Newton's third law:
T_AonS = T_SonA    (2), and
T_BonS = T_SonB    (3)

Using (1), yields:
T_SonA = T_SonB

T_SonA = T_SonB



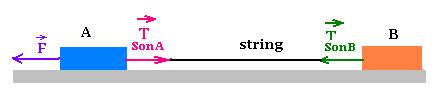
The two forces T_AonS = T_SonB act as an action-reaction pair, and we can even omit the string.

If the string is massless, it can be omitted, and: T_SonA = T_SonB

2. Example:

Let's consider two blocks A and B, connected by a massless string, and pulled across a frictionless table toward left by a force F exerted toward left on A. We consider the mass of B is larger than the mass of A. How about the tensions on the strings?

If the string is massless, then: T_BonA = T_AonB

The net force of the system (two blocks + two strings) is oriented to the left, therefore, the two blocks accelerate to the left.

For the block blocks A, Newton's second law is written as follows:
T_FonA - T_BonA = m_A a

Therefore
T_FonA = T_BonA + m_A a
and
T_FonA > T_BonA

For the block blocks B, Newton's second law is written as follows:
T_AonB = m_B a



T_FonA > T_BonA