Linear
optimization
Optimisation
linéaire
Avec Solveur d'Excel
MS Office
© The scientific sentence. 2010
| Mathematics 2: Linear optimization
Examples
Jean pants

A plant produces two kinds of jean pants : Jean A and Jean B.
A made jean pants passes through three machines:
Machine M1: cut the fabric. It operates at most 5 hours,
Machine M2: sew the pants. It operates at most 4 hours,
Machine M3: install rivets. It operates at most 2 hours.
• Jean A:
It costs 50.00 $.
The Machine M1 spends 5 mn to cut its fabric, the
Machine M2 spends 6 mn to sew the pants, and the
Machine M3 spends 8 mn to install special rivets.
• Jean B:
It costs 30.00 $.
The Machine M1 spends 3 mn to cut its fabric, the
Machine M2 spends 5 mn to sew the pants, and the
Machine M3 spends 2 mn to install rivets.
How many jean pants the factory should produce
to maximize its profit?
Solution
Set the unknown:
x is the number of jean pants of kind A
y is the number of jean pants of kind B
The operation time (in mn) for the
three machines:
M1: 5 x + 3 y ≤ 5 x 60 = 300
M2: 6 x + 5 y ≤ 4 x 60 = 240
M3: 8 x + 2 y ≤ 2 x 60 = 120
The equivalent equations are:
M1: y = - (5/3) x + 100
M2: y = - (6/5) x + 48
M3: y = - 4 x + 60
Their graph is:
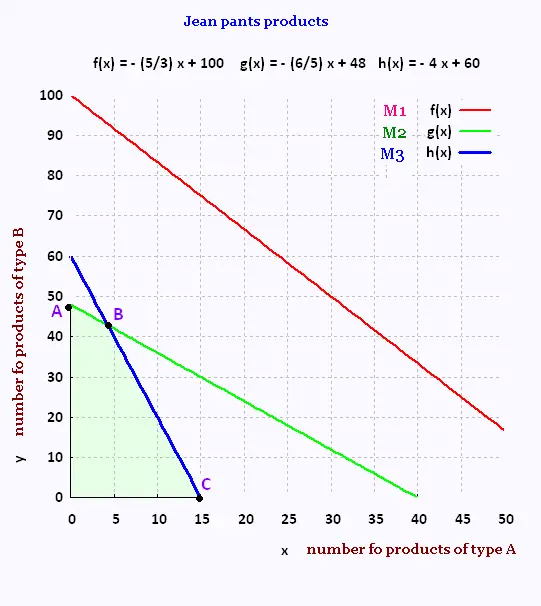
Function objective Z = 50 x + 30 y Maximum
| Vertex | Z ($) |
| O(0, 0) | 0 |
| A(0, 48) | 1440 |
| B(4.3, 42.86) → B(4, 42) | 1505 |
| C(15,0) | 750 |
To obtain the maximum profit which is 1505 $, the factory would produce 4 jean pants of kind A and 42 jean pants of kind B.
|
|