Mathematics
functions of
several variables
functions of
several variables
Partial derivatives
Differential
Linear approximation
Error calculation
Extrema of a function
© The scientific sentence. 2010
|
Calculus I:
functions of several variables
linear approximation
Approximation of a function of a single
variable
Approximation of a function
of a single variable
Once again we begin a chapter by recalling the
definition of the derivative of a function in a
single variable.
Definition
f: D → R
x → f (x)
We say that f is differentiable in x and of f'(x) is its
derivative when the next limit is defned, that is
the limit exists and it is not + ∞ or - ∞.
f'(x) = lim f(x + δx) - f (x)
δx → 0
Note :
Traditionally when we define the derivative of a function
in a theoritical point of view, the small number that
tends to 0 is noted h. When performing a calculation
of error, we use as notation δx instead of h.
Since we have a equality when δx tends to 0, we
deduct the following approximation
Proposition 1
f'(x) ≈ [f(x + δx) - f (x)]/δx
Exercise 1
Using the following table of values, give an
approximation of f'(3).
x 1.5 2 3 3.2 4 5
f(x) 6 10 12.5 15.6 20 25
Solution:
We apply the preceding formula with x = 3 and
δx = 0.2. We obtain:
f'(3) ≈ [f(3 + 0.2) - f (3)]/0.2 =
[f(3.2) - f (3)]/0.2 = (15.6 - 12.5)/0.2 = 15.5
f'(3) ≈ 15.5
Since
f'(x) ≈ [f(x + δx) - f (x)]/δx
we have
f'(x) δx ≈ f(x + δx) - f (x)
and therefore another way of writing the previous
approximation is:
Proposition 2
Affine approximation of a function of a variable).
f(x + δx) ≈ f'(x) δx + f (x)
Graphical interpretation
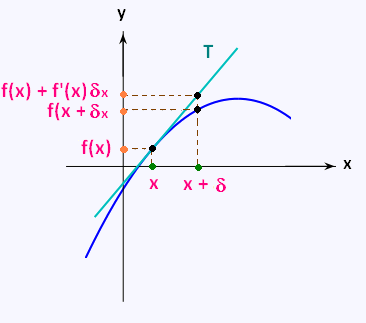
T is the tangent of f in x. With our notations
f'(x) δx + f (x) represents
the ordinate of the point of T of abscissa x + δx.
It is therefore natural to say that f(x + δx) and
f'(x) δx + f (x) are very close.
Exercise 2
Without calculator give an approximate value of
√2.05.
Solution:
We consider the following function f:
f: R+ → R+
x → √x
We are therefore looking for an approximation
of f(2.05).
We put: x = 1, dx = 0, 05.
We get: √2.05 = f (2.05) � f(2) + f'(2) x 0, 05.
We have: f (2) = 1.41
f'(x) = 1/(2√x), so f'(2) = 1/2x1.41 = 1/2.82.
Hence: √2.05 � 1.41 + 1/2.82 x 0.05 =
1.41 + 0.0177 = 1.4277.
Conclusion: √2.05 � 1.4277.
Note: The exact value of √2.05 is 1.4318 ...
|
|