Functionals:
Variational Methods
Funtional

1. Functional Concept

The functional is a generalization of the function. Rather than to depend on one (or more) variable, the functional depends on one (or more) function which depends on one (or more) variable. It is, therefore, a function function.

Let's consider a function y(x) that depends on a variable x and a function f of three not independant variables f(y(x), y'(x), x), where y'(x) = dy/dx .

The function y(x) is known, thus the function f takes a determined value for a given value of x. The dependency of f on the derivatve y'(x) occurs very often, mainly in Mechanics.

Note that the function f depends on x explicitly, but also implicitly through y(x) and y'(x). The total derivative of f is:

df = (∂f/∂y) dy + (∂f/∂y') dy' + (∂f/∂x) dx . Therefore:
df/dx = (∂f/∂y) y' + (∂f/∂y') y" + (∂f/∂x)

df/dx = (∂f/∂x) + (∂f/∂y) y' + (∂f/∂y') y"

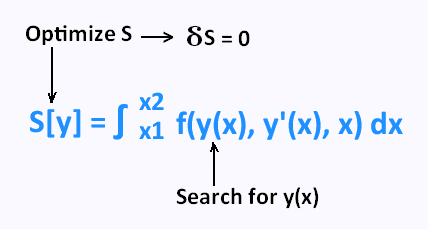
Let's define the functional S[y] by the integral:

S[y] = ∫ _x1^x2 f(y(x), y'(x), x) dx

Since the integral is definite, the functional S[y] est then evaluated; that is, it is a number.

Explicitly and before evaluating the integral, S[y ]depends on y(x)]. Using the variational method approach will allow us to determine the function y(x) that optimizes (minimize) the functional S[y], given that y(x1) and y(x2) are known.

2. The basic purpose

The basic purpose of the calculus of variations is to determine the function that extremizes a functional. In general, there can be more than one independent variable and the integrand can depend on several functions and their higher derivatives.