Calculus of Variations:
Functionals
Principle of Least Action
Pendulum

Pendulum

In this example, we will use the Lagrangian formulalism to determine the equation of a simple pendulum.



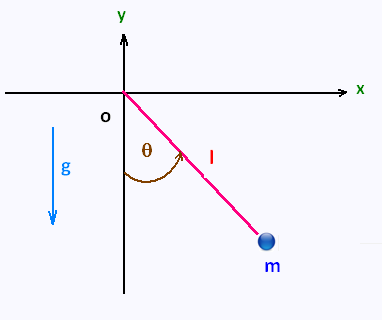
Let's consider a pendulum composed of an object of mass m and a massless string of length l in a constant gravitational field with acceleration g.

The motion of the pendulum is two-dimensional. It can be reduced to the a one-dimentional motion by using a single generalized coordinate which is the angle θ measured from the negative y-axis, such the position of the object is given as:

x (θ) = l sin θ
y (θ) = - l cos θ

with associated velocity components

x'(θ, θ') = l cos(θ) θ'
y'(θ, θ') = l sin(θ) θ'

Hence, the kinetic energy of the pendulum is

KE = (m/2) (x'² + y'²) = ml² θ'²/2.

KE = (ml²/2) θ'²

and choosing the zero potential energy point when θ = 0, the gravitational potential energy is PE = mgl(1 - cos θ).

PE = mgl(1 - cos θ)

The Lagrangian L = KE - PE is, therefore, written as

L(θ,θ') = (ml²/2)θ' ² - mgl(1 - cos θ)

and the Euler-Lagrange equation for θ is

∂L/∂θ' = ml²θ'. So

d(∂L/∂)/dt = ml²θ"
∂L/∂θ = - mgl sin θ

Therefore

ml² θ" = - mgl sin θ

or

θ" + (g/l) sin θ = 0

θ" + (g/l) sin θ = 0

That is the differential equation of a simple pendulum of period T = 2π √(l/g)