Optical instruments: the optics of an eye

1. Introduction:

A pair of glasses or contact lenses correct a faulty eyesight to produce normal vision.
The fundamental elements of an eye are illustarted in the following figure:

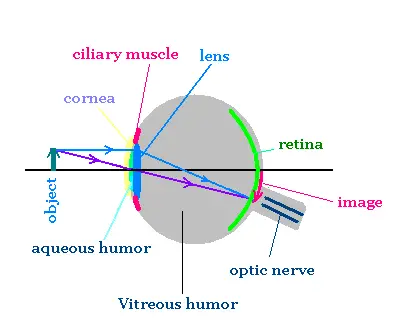
The eye is an optical instrument. The retina contains millions of rods and cones that, when simulated by light, send electrical impulses along to the optic nerve to the brain.
The most part of refrated light needed to produce an image occurs at the air-cornea interface. The index of refraction of air is 1.00 and the index of recraction of the cornea is about 1.38. At the aqueous humor, the index of recraction is 1.33, next the lens has an index of recraction of 1.40, and the vitreous humor 1.34. The lens contributes for only about a quarter pf the total refraction.
Altering the shape of the lens with the ciliary muscles, changes its focal length. When we see distant objects, the ciliary muscles are relaxed and the lens is relatively flat and causes a little refraction, then le focal length is at its greatest. When we view nearby objects, the ciliary muscles are tensed to give the lens more curvature, and the lens shortens its focal length, then more refraction. The process of changing the shape of the lens and hence adjusting its focal length is called accommodation.
A normal human eye produces sharp images of objects between about 25 cm and infinity. A differente situation is the case of visual defects or a reduction in focusing ability gotten with advancing age.
The three most common problems are nearsightedness, farsightedness, or astigmatism. The nearsightedness is the ability to focus on nearby objects, the farsightedness the ability to focus on far away objects, and the astigmatism is the defect, in which the eye is unable to sharply focus an entire image at any distance.

2. near and far point

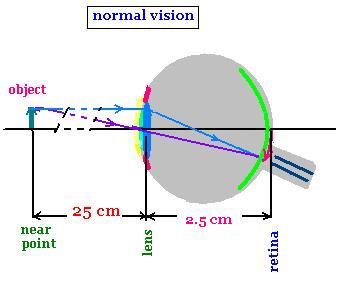
There is alimit on how the eye can focus. An object closer to the eye than a certain point called near point appears blurry. For young people, it is about 25 cm. It increases with age, and can be about 40 cm for people of age 40. It becomes about 500 cm in later years.
The far point is the greatest distance from the eye an object can be and still remains on focus. As we see stars, the normal far point is infinity. For the intensity of the light received by the eye, the iris plays the role of a diaphragm that contracts in bright light and dilates in dim light.

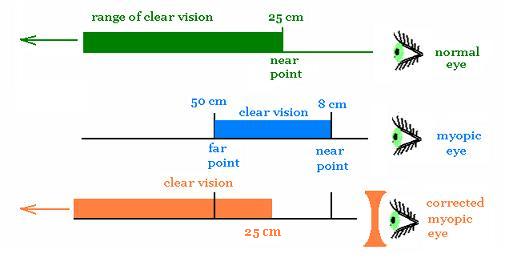
3. Nearsightedness

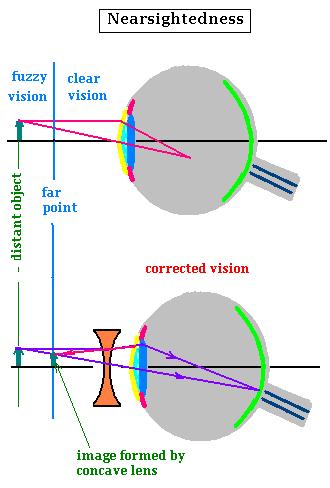
For a normal vision (clear vision from 25 cm to infinity), when the ciliary muscles are relaxed, an object at infinity is in focus. For a near-sighted (myopic), the relaxed eye focuses only within a finite distance from a certain point toward the eye , this point is called the far point. The near objects are focused, whereas objects beyond the far point are fuzzy (blured). In this case the eye converges the light in too short distance. To correct this excess of convegence effect, we need a diverging (concave) lens, placed in front of the aye, to make images fall again on the retina. At this effect, a blurry object at the infinity will be viewed a the related far point. The concave lens will produce the image of an object at infinity (distant object) at the myopic's far point, therefore, the relaxed nearsighted relaxed eye can now focus on the object.

Example: Extended vision A nearsighted has a far-point at 353 cm from the eye. If correcting glasses (thin diverging concave lenses) are set at 3.00 cm from the eye. What is the focal length of these lenses that will allow focusing on distant object.
The image of the distant object will be placed at the far-point = - q
distant point = infinity. According to the the Gaussian lens formula:
1/f = 1/p + 1/(- q) gives:
1/f = 0 - 1/q = - 1/(353 - 3.00) = - 1/350 (cm^-1)

4. Farsightedness

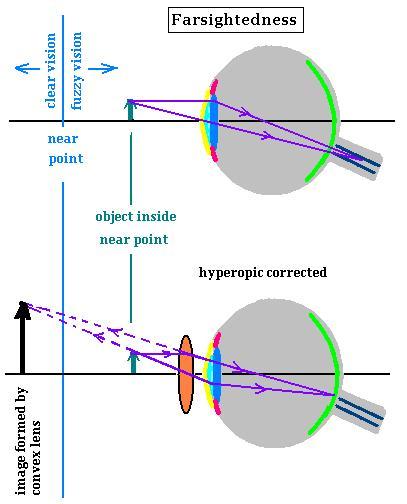
For a normal vision (clear vision from near-point 25 cm to infinity), when the ciliary muscles are relaxed, an object at the near point is in focus. For a far-sighted (hyperopic), the relaxed eye focuses only within a finite distance from a certain point, called the near point toward infinity. The near or closer objects are blurry, whereas objects beyond the near point are focused. The near-point for an hyperopic if much farther from the eye than the near-point's normal person, as a result, a farsighted person is unable to read clearly. In this case the eye converges the light in too long distance. Rays from an object inside the near point are brought to a focus behind the retina.
To correct this insufficiency of convegence effect, we need a converging (convex) lens, placed in front of the aye, to make images fall again on the retina. At this effect, a blurry object inside the near-point will be viewed beyond this near point where the vision is clear. The convex lens will produce the image of an object inside the near-point (near object) beyond the hyperopic's near point, therefore, the relaxed farsighted relaxed eye can now focus on the object with ease.

Example: Far sight better vision
A farsighted person wears glasses at 2.00 cm from his eyes, enabling him to read a book held at a distance 25.00 cm from his eyes. His near-point is 60.00 cm.
His glasses are converging convex lenses of focal distance f. We have:
1/f = 1/p + 1/q = 1/(25 - 2) + 1/(- (60 - 2)) = 1/23 - 1/58 = 0.026
Therefore f = 38.11 cm
The refractive power is 1/f (in m) = 2.6 diopters

5. Astigmatism

Astigmatism is usually produced by an asymmetry of the cornea which causes the effective focal length of the eye to behave differently for rays in the horizontal and vertical planes. A lens in the form of a cylinder section can be used to correct astigmatism.