Optical instrument Resolution :
1. Definition:
Theresolution is an important property of any optical
instrument, such as a microscope, a telescope, a camera,
or an eye.
The resolution of a certain optical device
is its ability to show two points apart
as separate points. In other words, it is the measure of
its ability to produce separate images of two adjacent points
objects. It is also called a resolving power
of the device. For example, if the resolution of a device is
equal to Re, and the two points are separated by S. If
S < Re then the two points will be blended and seen blured,
if S > Re, then the two points will be seen separated as really
they are.
2. Expression of the resolution
We have seen, with the Fraunhofer diffraction by a circular
aperture, the expression of the angular position θ1 related to Airy disk
(the first dark ring surrounding the bright central maximum) is
sin θ1 = 1.22 λ/d
λ is the wavelength of the used light in the device,
and d is the diameter of the circular aperture of the device.
θ1 is small, so: θ1 = 1.22 λ/d
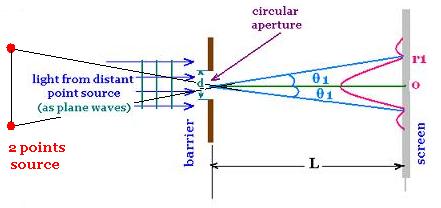
We have:
r1 = L θ1 = 1.22 λL/d
The central maximum is more spread out for longer wavelengths and for
smaller apertures.
For an optical instrument, the circular aperture is the lens
(for a microscope, a camera) or the entrance mirror (for
a telescope). The screen is an eye or detector.
Two points source of light (as stars for a telescope) produce
two images that are diffraction patterns with no interference
seen at the distance L (>> d) from the circular aperture.
The angle separation of the two points source from the eye (or detector)
must allow to show two points, not one. For this, the resolution is defined
by a criterion. We use the Rayleigh criterion. According to this
criterion, two images are just resolved if the center of the
central maximum of one pattern falls on the first dark ring of the other.
Therefore,
Two point objects separated by an angle Δθ are
clearly resolved when Δθ > ΔθR
ΔθR is the limiting angle of resolution
for an optical instrument with an aperture diameter d receiving
a light of wavelength λ. We have:
ΔθR = 1.22 λ/d
in radian (rad)
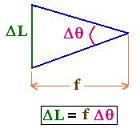For a microscope, the distance L is close to the focal length
f of the objective, the spatial resolution is:
r1 = θ1 = 1.22 λL/d, or
Δl = 1.22 f λ/d
For a telescope, the aperture diameter "D" of its objective is large
(about 5 meters), then we can take 1.22 = 1, so
ΔθR = λ/D
3. Electronic microscope:
Instead of using light, we use electrons. We know that
they behave as waves according to De Broglie formula:
λ = h/p
h is the Planck's constant, p the magnitude of the
linear momentum, and λ the associated wavelength
for the electron.
If E is the kinetic energy of the electron, E = mv2/2
Then
p = mv = m (2E/m)1/2 = (2mE)1/2
Using the De Broglie relationship p = h/λ yields:
λ = h/p = h/(2mE)1/2
Therefore, the angular resolution power is:
ΔθR = 1.22 λ/d = 1.22 h/d(2mE)1/2
h (Planck's constant) = 6.63 × 10-34 m2 kg/s
m (electron mass) = 9.11 × 10-31 kilograms
1 eV (electron volt) = 1.60 x 10-19 joules
1 cm = 1.0 x 10-2 m
Then:
ΔθR = 1.22 h/d(2mE)1/2 =
1.22 x 6.63 × 10-34/(2m)1/2 d(E)1/2
= 1.22 x 6.63 × 10-34/(2 x 9.11 × 10-31 x 1.60 x 10-19)1/2 x 1.0 x 10-2 d(E)1/2 = 1.5 x 10-9/d E1/2
ΔθR = 1.5 x 10-9/dE1/2
d in m, and E in eV
ΔθR = 1.5 x 10-7/dE1/2
d in cm, and E in eV
4. Comparison: ΔθR(light) and ΔθR(electron)
If E = 1.0 eV , and d = 1.0 cm
ΔθR = 1.5 x 10-7 =
0.15 x 10-6 rad = 0.15 µn;rad.
If we consider a yellow light with a wavelength of 580 nm,
the resolution will be:
ΔθR = 1.22 x 580x -9/1.0 x -2
708 x -7 rad = 71 µrad
The limiting resolution of an electron microscope is
much higher than that of an optical microscope
Remark:
For an electron, the wavelength is λ = h/(2mE)1/2 =
6.63 × 10-34/(2x 9.11 × 10-31x 1.60 x 10-19E)1/2
= 1.23 x 10-9 /E1/2
Therefore:
λelectron = 1.23 nm/E1/2
E in eV
|
