I. Classical Rutherford's differential cross section:

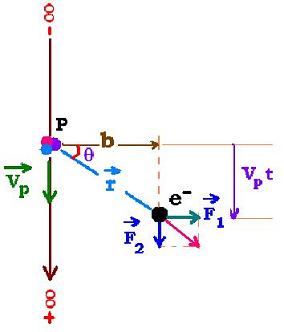
Let's consider a collision between two particles P_p and P_c with charges Z_p and Z_c respectively. The related magnitude Coulomb's force is:
F_pc = (1/4πε_o)Z_pZ_c e²/r² Where r is the distance between P_p and P_c at the time t. The perpendicular force to the P_p direction is the only important regarding the Coulomb's force. F_p becomes F = F_p cos θ = (1/4πε_o)Z_pZ_c e² cos θ/r².
The transferred moment to the particle P_c by P_p is: P = ∫Fdt.
r sin θ = V_p t → V_p dt = dr sinθ + b dθ.
V_p is the velocity of the P_p particle and b the impact parameter.
dr = b (sin θ/cos² θ) dθ → V_p dt = (1 + tg²Î¸) dθ.
Then P = (1/4πε_o)Z_pZ_c e² ∫ cos θ/r² dt
= (1/4πε_o)Z_pZ_c e² ∫ cos θ/r² dt
= (1/4πε_o)Z_pZ_c e² (1/V_pb) ∫ cos θ dθ [- Ï€/2: +Ï€/2].
P = Z_pZ_c e²/2πε_o V_pb    (1)

We consider the target particle P_c, that gains the transferred moment, is an electron of an atom of mass m_e. From the relationship (1), its energy becomes:
T = P²/2m_e = Z_p² e⁴/2m_e (2πε_o V_pb)²    (2)
We solve the relationship (2) for b and derive over dT, we find the differential cross section dσ related to the collision :

dσ = 2Ï€bdb = (2Ï€Z_p² e_o⁴/m_ec² β²) dT/T²    (3)

Where e_o² = e²/4πε_o and β = V_p/C. c is the speed of light in the vacuum and e is the electron's charge = 1.6 10^-19 Coulomb.
That is the differential cross section for that the projectile, with a charge Z_p and velocity V_p, collides with an electron target of mass m_e and transferts to this electron a kinetic energy in the range T and T + dT.

II: Binary Elastic collision

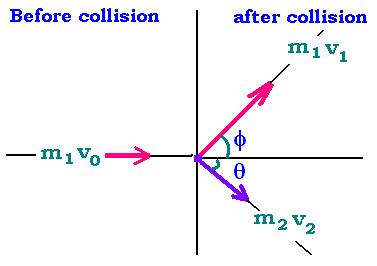
Particle 1: mass m₁.
Particle 2: mass m₂.

The conservation of energy and momentum before and after colision gives the following formulas:
m₁v₀ = m₁v₁ cosφ + m₂v₂ cosθ    (1)
m₁v₁ sinφ = m₂v₂ sinθ    (2)
m₀v₀² = m₁ v₁² + m₂ v₂²    (3)

We square (1) and (2) and make the difference:
m₁² v₁² = m₂² v₂² sin² θ + (m₁v₀ - m₂v₂ cos θ)².
With the relationship (3), we find:
v₂ = 2 ((m₁/(m₁ + m₂)) v₀ cosθ.

The kinetic energy tranferred to the second particle is: T = (1/2)m₂v₂²; then:
We have:
T = 2 m₁² m₂ v₀² cos²Î¸/(m₁ + m₂)²

cos² θ = T/T_max
T_max = 2 m₁² m₂ v₀²/(m₁ + m₂)²

T_max is the maximum energy that the particle 1 can transfert for the particle 2.

In the case of the particle 1 is more heavier than the second (m₁ >> m₂), we can write:
T_max = 2 m₂ v₀².

T_max (proton → electron) = 2 m_e V_p²

III: The simplest approach: The Katz's model:

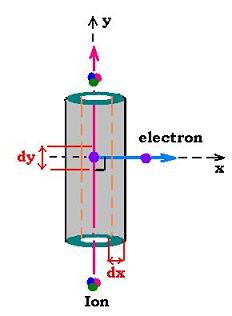
In this model, an electron is ejected at low energy; that is cos &thèta; is around zero degree.
If dn is the number of electrons ejected by the incident ion per unit length dy, and N is the number of electrons per unit volume, we have:
dn = N dσ = (C Z_p²/β²) dT/T²
C = 2Ï€N e_o⁴/m_e c².
The distance-energy relationship:
We consider the following one:

x = k T(x)    (1)

From this relationship (1), we have x_max = kT_max and dT/T = k dx/x²

Where x is the diatance from the path of the incident ion, T(x) is the remaining enrgy of the electron at x, and k is a constant.
From the relationship (1), we get: dT/T² = k dx/x².

Only the electrons that have a kinetic energy T(x) or greater can arrive at x and traverse the layer dx, the number of those electrons is n(x) = ∫ dn(x) dx.
n(x) = (K Z_p²/β²) &int dT/T².
= (C Z_p²/β²) [1/T(x) - 1/T_max]
n(x) = k(C Z_p²/β²) [1/x - 1/x_max]    (2)

In the layer dx of volume dV = 2Ï€ x dx dy, where dy is the unit length of the ion's path, every electron deposits the enrgy dT = dx/k; forthermore n(x) electrons crossing the layer dx will deposit in the volume dV the energy n(x) dT = n(x) dx/k.
The dose defined as the enery deposoited by volume unit is then written as:
D(x,βC_p) = (n(x) dx/k)/dV = (n(x) dx/k)/(2π x dx dy). From the relationship (2), we can write:
= D(x,βC_p) = k(C Z_p²/β²) [1/x - 1/x_max] dx/k /(2Ï€ x dx dy)
= (C Z_p²/β²) [1/x - 1/x_max] /(2Ï€ x dy)
Generally, x_max is often greater than x, we have then, per unit length of the incomming ion:

D(x,&beta,Z_p) = [C Z_p²/2Ï€ β²] 1/x²    (3)


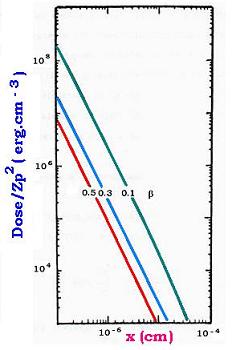
For 100 MeV protons in water, we have:
N = 3.35 x 10^-23
C = 8.50 (e^-) eV/µm
Energy of proton E_p = (1/2) M_pV_p²
= (1/2) M_pc² β² = (1/2) 938.272 β² MeV = 469.136 β² MeV
E_p = 469.136 β² MeV
With E_p = 100 MeV → β = 0.462
D(x,100 meV) = [8.50 eV/µm /2Ï€ β²] 1/x² = 63.50 1/x² keV/cm³

D(x,100 MeV) = 63.50/x² keV.cm^{- 3}

In erg unit, we have:
D(x,100 meV) = 1.018 10 ^{- 7}/ x² erg . cm^-3