2. Macrostates & Microstates
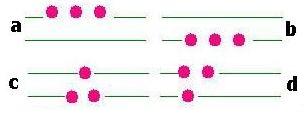
Let's consider the following example:
We have 3 particles and 2 energy levels. If the particles are indistinguishable,
we have then 4 configurations.
If the particles are distinguishable, we have 8 configurations.
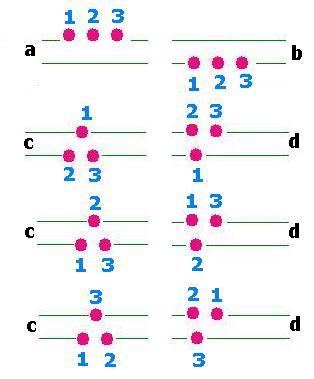
The number of macrostates is
4: Ω(a), Ω(b),Ω(c),Ω(d).
The number of microstates is
1 for Ω(a) and for Ω(b), and 3 for Ω(c) and Ω(d).
Remark
In the case of indistinguishable particles, every macrostate has no microstate. Forethermore:
Ω(a) = Ω(b) = Ω(c) = Ω(d) = 1. In other words, the macrostate is the
microstate.
Now, let's fix the energy of this system with three particle to be E. Particles can occupy
the two energy levels ni (i = 1, 2).
If Ei (i = 1, 2) is the value of the energy associated to the level i, then:
E = Σ ni [i = 1, 2].
As the total number of particles is already fixed to be N (here N = 3), if the number of
levels is n; we have:
E = Σ niEi
N = Σ ni
[i: 1 → n]
Now, having ni particles in each livel "i", that determines the macrostate for
the system, how many microstates do we have for this macrostate?
If n1 is the number of particles that lodge in the level "1", the number of ways
to have this occupation is the number of combinations for n1 particles
among N particles; that is :
C1 = C(n1, N) = N!/n1!(N - n1)!
Once this case is set; it remains (N - ni) particles to consider.
If n2 is the number of particles that lodge in the level "2", the number of ways
to have this occupation is the number of combinations for n2 particles
among (N - n1) particles; that is:
C2 = C(n2, (N - n1)) = (N - n1)!/n2!((N - n1) - n2!
...
Finaly, if the last level contains nn particles = N - n1 - n2 - ... - nn - 1, then
the numbers of ways to distribute hem in within the last level "n" is Cn = C(nn, nn) = 1.
In total, the number of ways we have is the product of all the combinations. That is:
C1 x C2 x ... x Cn = ΠCi [i: 1 → n]
= N!/n1!(N - n1)! x (N - n1)!/n2!((N - n1) - n2! x ... x 1. = N!/n1 x n2 x ... x 0!= N!/Πni [i: 1 → n]
The number of ways to distribute N particles over
n levels containing each ni particles is Ω = N!/Πni [i: 1 → n]]
In the case of a level "i" has a degeneracy gi; that is the number gi
subshells, we can place ni particles within by gini ways; Thus:
The number of ways to distribute N particles over
n levels containing each ni particles; with a degeneracy gi for
the level "i" is Ω = N! Π(gini/ni) [i: 1 → n]]
The configuration (n1, n2, ..., ni, ..., nn) represents a macrostate
wich has Ω = N! Π(gini/ni) microstates
3. Maxwell-Boltzmann distribution
Suppose that we toss up two dimes. The ways that we an have are:
(heads, heads); (tails, tails); (heads, tails) or (tails, heads) because we distinguish the pieces. The probability for each case is 1/4. If instead we have two balls, red and yellow, then indistinguishable, we will have the case: (red, red); (yellow, yellow); (red, yellow) = (yelolow, red). The probability for each case is 1/4, 1/4, 2/4. We say that the last case is most probable.
We are interested in the most probable case. To find the most probable case from the expression of Ω we derive it with respect to the number of particles ni and zero if in order to find the extrema which give the most probable configuration.
From the expression of Ω itself, we can not go further. Taking the its Neperian logarithm "ln" will simplify graetly the issues.
Ω = N!Π (gini)/ni!, thus:
ln (Ω)= ln N! + Σ [niln gi - ln ni!]
Using the Stirling's approximation
ln x! = x ln x - x , we have:
ln (Ω)= ln N - N + Σ [niln gi - ni ln ni + ni] =
ln N + Σ [ni][ln gi - ln ni] = ln N + Σ [ni][ln (gi/ni)]
Thus:
ln (Ω) = Σ [(dni)(ln(gi/ni)) - dni]
ln N! = Σ ln x [x: 1 → N] = ∫ ln x dx [x: 1 → N]
= xln x - x [x: 1 → N] = Nln N - N + 1 . Neglecting 1, we can write: ln n! = nln N - N.
Example: For N = 100, we have ln N! = 364 and Nln N - N = 360; an error ≈ 1% !
We have the following constraints:
N = Σ ni
E = Σ niEi
Or :
N - Σ ni = 0
E - Σ niEi = 0
If ∂ln(Ω)/∂ni = 0, a linear combination with the constraints gives
also zero (method using constants called Lagrange multipliers).
Let's define then a new function F as follows:
F(ni) = ln(Ω) - λ(N - Σ ni) + β(E - Σ niEi)
= NlnN - Σniln ni - λ(N - Σ ni) + β(E - Σ niEi)
To find the extrema, Its derivative is :
∂F(ni)/∂ni = 0
That is:
- 1 - ln ni + λ - β Ei = 0
Let's set: λ - 1 = α; then:
- ln ni + α - β Ei = 0 .
Thus:
ni = exp[ α - β Ei];
and
N = Σ ni = Σ exp[ α - β Ei]
= exp[ α] Σ exp[ - β Ei]
exp[ α] = N/Σ exp[ - β Ei]
Let's set:
Z = Σ exp[- β Ei]
called the partition function.
It follows that: exp[ α] = N/Z. We find the
Maxwell-Boltzmann distribution:
ni = Nexp(-βEi)/Σexp(-βEi)
= Nexp(- βEi)/Z