A sigle wave
Superposition of waves
© The scientific sentence. 2010
|
Doppler effect
1. Moving source forward an observer at rest

The source S is moving at right at the speed Vs in the frame of
the observer at rest. At the precise time T, which is the period of the wave;
this wave that was emitted from So reaches the observer. The source at
this time is not located at the position So, that is at the distance Ls,
but It was moved and now It is located at the distance L's. For the
observer, It appears that the wave was originated from the position S
taking T' as time to arrive at its position. If Vwis the speed of the
wave, we can write:
L's = Vw. T'
We have:
Ls = Vw. T
And:
L's = Ls + Vs. T
Thus:
Vw. T '= Vw. T +Vs. T
Then:
T' = T . (Vw+ Vs)/Vw
With ƒ = 1/T, we have:
ƒ' = ƒ[Vw/(Vw+ Vs)]
ƒobs = ƒ . Vw/(Vw+ Vs)
Where ƒ' is the apparent frequency
for the Observer, and ƒ is the real frequency of the wave.
If the source is stationary (Vs = 0), then ƒ' = ƒ' and
T' = T, that is the observer gets waves at every real period.
2. Moving source toward an observer at rest
 Next, the observer is always at rest and the source
S is moving at a speed Vs towards this observer. The wave propagates
at the speed Vwin all directions and towards the observer of course.
When this observer receives the wave, at this precise time, the
source is not located at the distance Ls but at L's. For the observer, the
period of the wave is T's. And at this precise time, the wave has
travelled Ls = Vw. T , where T is the real period .
T's is the apparent period.
Next, the observer is always at rest and the source
S is moving at a speed Vs towards this observer. The wave propagates
at the speed Vwin all directions and towards the observer of course.
When this observer receives the wave, at this precise time, the
source is not located at the distance Ls but at L's. For the observer, the
period of the wave is T's. And at this precise time, the wave has
travelled Ls = Vw. T , where T is the real period .
T's is the apparent period.
We can write:
L's = Ls - Vs . T
Because Ls = Vw. T
and L's = Vw. T's
Thus :
Vw. T's = Vw. T - Vs . T = (Vw- Vs) . T
Or:
1/T's = Vw/(Vw- Vs) (1/T)
ƒ' = Vw/(Vw- Vs) ƒ
ƒobs = ƒ . Vw/(Vw- Vs)
ƒobs is the apparent frequency.
3. Moving source and observer
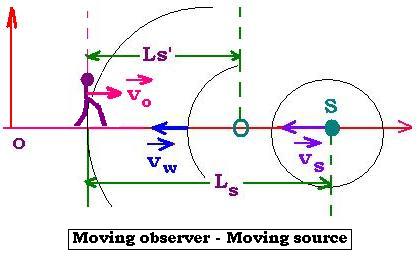
Now, let's consider that the observer is moving
at a speed Vo and the sourse is moving at a speed Vs,
oppraching the observer, that is in the opposite direction.
The wave is always moving at the velocity Vw.
This case is like the last one except that the observer
is moving. Then insted of L's = Vw. T' , we have:
L's = (Vw + Vo) . T'
Thus:
(Vw + Vo) . T' = (Vw- Vs) . T
Hence:
ƒ'=(Vw+Vo)/(Vw-Vs)ƒ
ƒobs = ƒ . (Vw+Vo)/(Vw-Vs)
4. Moving source and observer : Relativistic case
In the relativistic case, the time is streched, that is the time
T becomes γT, where
γ = 1/[1- (Vs/Vw)2]1/2.
Hence
the frequency (1/T) is changed into: 1/γT. That is:
ƒ' (Relativistic) = ƒ'(Classic)/γ
For the latter case, we have:
ƒ'(Relativistic) = (Vw+Vo)/(Vw-Vs)ƒ/γ = (Vw+Vo)/(Vw-Vs) [1- (Vs/Vw)2]1/2 ƒ
= ƒ . [(Vw+Vs)/(Vw-Vs)]1/2 . (Vw+Vo)/Vw.
ƒ' = ƒ . [(Vw+Vs)/(Vw-Vs)]1/2 . (Vw+Vo)/Vw
ƒobs = ƒ.[(Vw+Vs)/(Vw-Vs)]1/2 . (Vw+Vo)/Vw
In the case of the wave is light, Vw= c (speed of light in the vacuum = 3.10 8m/s)
Then :
ƒ' = ƒ . ((c + Vs)/(c - Vs))1/2 . (c + Vo)/c
In the case of the observer is at rest, V0 = 0, then:
ƒ' = ƒ . [(c + Vs)/(c - Vs)]1/2.
ƒ' = ƒ . (1/c)(c + Vo)/[1 - (Vo/c)2)]1/2 ≈ ƒ(1 + Vo/c)
ƒobs = ƒ (1/c)(c + Vo)/[1 - (Vo/c)2)]1/2 ≈ ƒ(1 + Vo/c)
©: The scientificsentence.net. 2007.
|
|
|